

Up: New times of minima
Subsections
The times of minima were measured at several observatories:

- Kryonerion Astronomical Station of the National Observatory
of Athens, Greece - 1.2 m telescope,

- Carter Observatory, New Zeeland - 0.6 m telescope,

- Ondrejov Observatory of the Czech Academy of Science,
Czech Republic - 0.65 m telescope,

- ESO La Silla Observatory, Chile - 0.5 m ESO telescope, and

- Wendelstein Observatory, University of Munich,
Germany - 0.8 m telescope.
The telescope in Ondrejov has been equipped with a CCD
camera, the other telescopes with photometers with photomultipliers.
The newly measured minima are given in Table 1. Their times were
determined by the Kwee-van Woerden (1956) method. Other published
times of minima have been used
in constructing the O-C graphs, and their table can be obtained by
e-mail from PM (mayer@mbox.cesnet.cz).
In all cases where primary and secondary minima were measured or
published, the phase of secondary minima is unrecognizable
from 0.5, i.e., the systems possess circular orbits.
The older photoelectric minima were collected by Mayer (1987).
It appears that a constant period suits all photoelectric minima.
Since the minimum time given in Table 1 is the one with highest
accuracy, we take it as the base for new ephemeris:
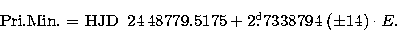
Note that rather unrealistic values for the orbital period are
given in GCVS (2 733849) and SAC (2
733849) and SAC (2 733826).
733826).
When older photographic minima are considered, it appears
that the period continues to shorten, see Fig. 1. The reason of
this shortening is unknown. Since light-time effect could be
responsible for it, we took several coudé CCD spectra of this
binary with the 2.2 m telescope of the German-Spanish Observatory
at Calar Alto, in order to find spectroscopic hints for the existence
of a third body. However, no third lines were found, i.e., the
luminosity of the eventual third body cannot be larger than about
5% of the integral light of the system in the blue spectral region.
From the deviations of the O-C data from linearity the minimum mass
of the eventual third body might be roughly estimated as about
1.5 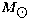 ; the expected luminosity of such a body is of course
well under sensitivity of any present spectroscopy.
; the expected luminosity of such a body is of course
well under sensitivity of any present spectroscopy.
![\begin{figure}
\includegraphics [width=8.5cm,clip=]{337aql.eps}\end{figure}](/articles/aas/full/1998/11/ds1452/Timg6.gif) |
Figure 1:
O-C graph for V337 Aql; plus signs denote photographic data,
circles photoelectric data |
The O-C column is calculated with the ephemeris by Bell et al. (1987):
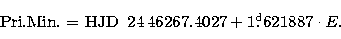
The scatter of published times of minima is large, see Fig. 2. In
this case lines of a third body were found on spectra taken by RL
with the coudé auxilliary telescope feeding the 3.6 m telescope coudé
spectrograph at ESO La Silla, and with the Calar Alto 2.2 m telescope
and its coudé spectrograph. This system will be thoroughly
discussed in a forthcoming paper by Lorenz et al. However, the
fast changes of O-C values are inexplicable by a light-time effect.
In Sect. 3 it is suggested that these changes might be connected with
stability of the light curve.
![\begin{figure}
\includegraphics [width=8.5cm,clip=]{1182aql.eps}\end{figure}](/articles/aas/full/1998/11/ds1452/Timg8.gif) |
Figure 2:
O-C diagram of V1182 Aql |
V1331 Aql is a detached early B-type binary, for which period and
minimum times were published by Lorenz et al. (1991). The O-C values
presented in Table 1 were calculated using the ephemeris given in
that paper:

UBV light curves were published by Lorenz et al. (1990).
A detailed study of this system by Lorenz et al., including
absolute dimensions derived from a recently established radial
velocity curve and the light curve analysis, is in preparation.
New times of minima of this well-studied three-body system
(Drechsel et al. 1994) should precise the period of the third
body; theory also asks for a long-period (335 years) change of the
orbital period (Mayer 1983). O-C values were calculated according
to the ephemeris
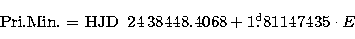
and phases in the third-body orbit according to
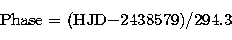
(see Mayer 1987). Two minima given in Table 1 fit the older values of
O-C (and the theoretical curve given in Fig. 3 of Mayer 1990)
very well, so no changes of periods in the orbit of the eclipsing
pair or in the orbit of the third body are apparent.
This eclipsing variable is a part of a multiple system (see
e.g. Morrison & Conti 1980). The period of this variable is very
close to 6 days, which means that minima can be measured well
only in a limited interval of geographic longitudes in a given
year. The observations published by now do not comprise any detailed
measurements of the deepest part of a minimum of the QZ Car light
curve. In Fig. 3 such measurements obtained at the
Carter Observatory are plotted. These measurements were reduced using
HD 93131 (a WR star, with V=6.50, B-V=-0.03 and U-B=-0.88) as the
comparison star. This star, used as the comparison star also in the
discovery paper by Walker & Marino (1972) however might be slightly
variable, the variability being of the order of 0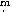 01; note that
Morrison & Conti recommend HD 93502. The time of
the minimum calculated from these measurements is the most precise
time known by now, so a new ephemeris is suggested (the period is
according to Mayer et al. 1992):
01; note that
Morrison & Conti recommend HD 93502. The time of
the minimum calculated from these measurements is the most precise
time known by now, so a new ephemeris is suggested (the period is
according to Mayer et al. 1992):
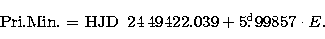
In the first part of the year 1998, the minima of QZ Car will be
observable in South America.
![\begin{figure}
\includegraphics [width=8.5cm,clip=]{qzcar.eps}\end{figure}](/articles/aas/full/1998/11/ds1452/Timg14.gif) |
Figure 3:
V magnitudes for QZ Car during a primary minimum |
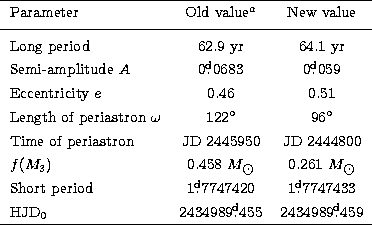
This bright detached eclipsing O-type binary, which is of special
interest because of the pronounced light-time effect
it displays, was thoroughly discussed by Drechsel et al. (1989).
Here, 10 new minima published by other authors, which
deviate systematically from the light-time effect curve as given in
Fig. 3 of that study, were used to
calculate a new set of the light-time effect parameters (see
Table 2). The light-time effect curve corresponding to these
parameters is shown in Fig. 4. The minima observed after
E=6000 approximately follow a linear ephemeris
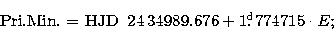
this ephemeris can be used to forecast minimum times in several
next years.
![\begin{figure}
\includegraphics [width=8.5cm,clip=]{ahcep.eps}\end{figure}](/articles/aas/full/1998/11/ds1452/Timg17.gif) |
Figure 4:
O-C diagram for AH Cep. The curve corresponds to the
light-time effect described by parameters given in Table 2;
plus signs are photographic, circles photoelectric data |
Except for the discovery paper (Hoffmeister 1943), this star was
never studied. In GCVS the spectral type is given as OB, however,
the source of this classification is unknown. There is a star No.
3268 in the catalogue Luminous Southern Stars
(Stephenson & Sanduleak 1971), with type OB(+)h, only 3 arcmin away; we used this
star as the comparison star. GSC numbers, J2000 coordinates
and magnitudes of both stars are:
AQ Cir: GSC 9015.0071, CPD -64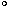 2941
2941
 -
-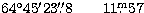
comparison: GSC 9015.0147, CPD -64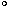 2939
2939
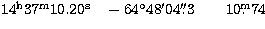 .
.
![\begin{figure}
\includegraphics [width=8.5cm,clip=]{aqcir.eps}\end{figure}](/articles/aas/full/1998/11/ds1452/Timg22.gif) |
Figure 5:
AQ Cir, measurements in B filter |
Hoffmeister gives 7 times of minimum light and period 0 57284
(as he remarks, the true period is probably twice as long). From
his original data it appears that the accuracy of the period is
about
57284
(as he remarks, the true period is probably twice as long). From
his original data it appears that the accuracy of the period is
about 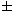 0
0 00002 ; this means that at present, the epoch number
is not known unambiguously. The large magnitude difference between
the variable and comparison given in GSC means that in the time of
exposition (JD 2446940.58) the variable had to be close to a minimum.
We measured a part of the light curve covering a phase range after
a minimum, and one can estimate when the minimum had appeared -
perhaps at about HJD 2449012.67
00002 ; this means that at present, the epoch number
is not known unambiguously. The large magnitude difference between
the variable and comparison given in GSC means that in the time of
exposition (JD 2446940.58) the variable had to be close to a minimum.
We measured a part of the light curve covering a phase range after
a minimum, and one can estimate when the minimum had appeared -
perhaps at about HJD 2449012.67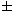 .05. Unfortunately, no
certain conclusion concerning the period can be reached, and further
measurements are necessary. We present our measurements in Fig. 5
in order to facilitate the determination of the period (note that the
GSC magnitudes correspond to V magnitudes; we measured B values).
.05. Unfortunately, no
certain conclusion concerning the period can be reached, and further
measurements are necessary. We present our measurements in Fig. 5
in order to facilitate the determination of the period (note that the
GSC magnitudes correspond to V magnitudes; we measured B values).
Older minima of V382 Cyg observed before the year 1990 are
listed by Mayer (1980) and MWTN. Several times of minima were
published recently, namely by Agerer (1991, 1992, 1993, 1994, 1996)
and by Agerer & Hubscher (1995); we have also measured three more.
The column O-C is calculated with ephemeris by Mayer et al. (1986):
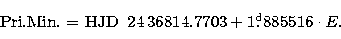
During the time interval covered by observations the period
has considerably lengthened (Mayer 1980). However, it appears that
the period changed last around E=4700, but then it has
been constant, so the presently valid ephemeris can be written as
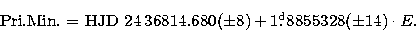
According to this ephemeris the column (O-C)1 of Table 1 has
been calculated and Fig. 6 drawn.
The photoelectric times of minima in the interval of epochs from 0
to 4700 fit also a linear ephemeris:
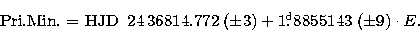
![\begin{figure}
\includegraphics [width=8.5cm,clip=]{382cyg.eps}\end{figure}](/articles/aas/full/1998/11/ds1452/Timg27.gif) |
Figure 6:
V382 Cyg, (O-C)1 diagram. Plus signs are photographic,
circles photoelectric data |


Up: New times of minima
Copyright The European Southern Observatory (ESO)
![]()
![]() ; the expected luminosity of such a body is of course
well under sensitivity of any present spectroscopy.
; the expected luminosity of such a body is of course
well under sensitivity of any present spectroscopy.
![]()
![]()
![]()
![]()
![]()
![]()
![\begin{figure}
\includegraphics [width=8.5cm,clip=]{ahcep.eps}\end{figure}](/articles/aas/full/1998/11/ds1452/Timg17.gif)
2941
-
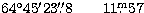
2939
.
![]() 57284
(as he remarks, the true period is probably twice as long). From
his original data it appears that the accuracy of the period is
about
57284
(as he remarks, the true period is probably twice as long). From
his original data it appears that the accuracy of the period is
about ![]() 0
0![]() 00002 ; this means that at present, the epoch number
is not known unambiguously. The large magnitude difference between
the variable and comparison given in GSC means that in the time of
exposition (JD 2446940.58) the variable had to be close to a minimum.
We measured a part of the light curve covering a phase range after
a minimum, and one can estimate when the minimum had appeared -
perhaps at about HJD 2449012.67
00002 ; this means that at present, the epoch number
is not known unambiguously. The large magnitude difference between
the variable and comparison given in GSC means that in the time of
exposition (JD 2446940.58) the variable had to be close to a minimum.
We measured a part of the light curve covering a phase range after
a minimum, and one can estimate when the minimum had appeared -
perhaps at about HJD 2449012.67![]() .05. Unfortunately, no
certain conclusion concerning the period can be reached, and further
measurements are necessary. We present our measurements in Fig. 5
in order to facilitate the determination of the period (note that the
GSC magnitudes correspond to V magnitudes; we measured B values).
.05. Unfortunately, no
certain conclusion concerning the period can be reached, and further
measurements are necessary. We present our measurements in Fig. 5
in order to facilitate the determination of the period (note that the
GSC magnitudes correspond to V magnitudes; we measured B values).
![]()
![]()
![]()