

Up: Oscillator strengths for As
Each atom is a many-body system which may be described by an
independent-particle-model. In this model, each electron moves
independently in an effective potential determined by the nucleus and
the other electrons. The potential for an electron in a neutral or
ionized atom is assumed to have the form
![\begin{displaymath}V({r}) = - \left( 2/{r} \right) \{(Z-\eta)
[H ({\rm e}^{{r}/{d}} -1)+1 ]^{-1}+\eta \}
\end{displaymath}](/articles/aas/full/2000/09/ds9354/img6.gif) |
(1) |
where Z is the atomic number, and 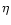 is the ionicity:
is the ionicity:
 ,
2, 3 for As I, As II, As III, respectively.
The quantity r is the electron-nucleus distance, and d, H are
adjustable parameters. The potential of equation (1) is
inserted into the radial Schrödinger equation, which is then
solved to obtain the energy eigenvalues and wave functions. The
parameters d, H are adjusted so as to obtain agreement between the
energy eigenvalues and the experimental single-particle energy levels.
This procedure and all necessary formulas may be found in a previous
article ([Ganas 1995]). The following values of the potential parameters
were obtained:
d = 1.4196,
H = 8.1745 for As I;
d =
4.7814,
H = 39.726 for As II;
d = 0.6094,
H = 3.0641 for
As III.
,
2, 3 for As I, As II, As III, respectively.
The quantity r is the electron-nucleus distance, and d, H are
adjustable parameters. The potential of equation (1) is
inserted into the radial Schrödinger equation, which is then
solved to obtain the energy eigenvalues and wave functions. The
parameters d, H are adjusted so as to obtain agreement between the
energy eigenvalues and the experimental single-particle energy levels.
This procedure and all necessary formulas may be found in a previous
article ([Ganas 1995]). The following values of the potential parameters
were obtained:
d = 1.4196,
H = 8.1745 for As I;
d =
4.7814,
H = 39.726 for As II;
d = 0.6094,
H = 3.0641 for
As III.
Representative energy levels are given in Table 1 for
As III. The computed levels and the experimental levels are in
good agreement, the discrepancy being less than 2% in every case
(except one).
Table 2:
Oscillator strengths, f, for the resonance transition
4p3(4S
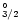 )
)
 4p2(3P)5s(4PJ) in As I, from the
present calculations, other calculations, and experiment
4p2(3P)5s(4PJ) in As I, from the
present calculations, other calculations, and experiment
|
J |
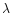 (Å) (Å) |
f (this |
f |
f (other |
| |
|
calculation) |
(experiment) |
calculations) |
| 1/2 |
1972.6 |
0.0532 |
 |
0.0584d |
| |
|
|
0.074b, 0.0734c |
0.06e, 0.03f |
| 3/2 |
1937.6 |
0.1064 |
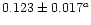 |
0.113d |
| |
|
|
0.14b, 0.139c |
0.11e, 0.06f |
| 5/2 |
1890.4 |
0.1596 |
0.21b, 0.214c |
0.161d, 0.16e |
- a
- Bengtsson et al. (1992): uses time-resolved laser spectroscopy.
- b
- Andersen et al. (1974): uses the beam-foil technique.
- c
- Lotrian et al. (1980): uses emission spectroscopy.
- d
- Verner et al. (1994): gives a critical review of f-values
from previous compilations.
- e
- Holmgren (1975): uses optimized Hartree-Fock-Slater
local exchange approximation and relativistic wave functions.
- f
- Lawrence (1967): uses an intermediate coupling theory.


Up: Oscillator strengths for As
Copyright The European Southern Observatory (ESO)